Although I designed the particulars of the construction of this model, the idea of a Sierpinski Tetrahedron is not original. It comes from one of the best-known fractals, the Sierpinski triangle.
Image borrowed from David Darling.
The Sierpinski triangle is constructed by taking three congruent triangles, and using them to form a larger triangle. Then we take two more copies of the larger triangle, and use them to form an even larger triangle. And repeat ad infinitum.
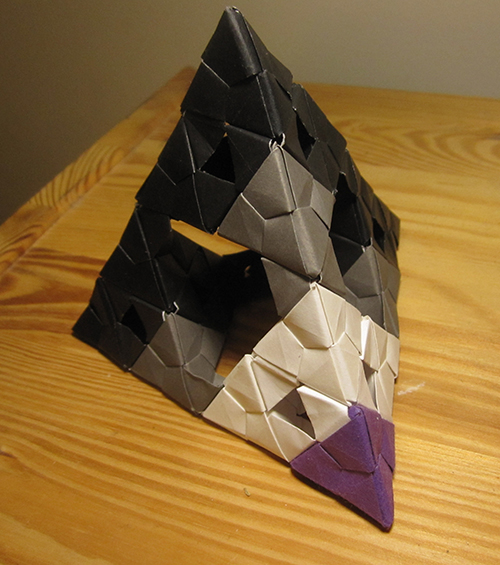
The sierpinski tetrahedron is a natural generalization, where you take four congruent tetrahedrons, and use them to form an even larger tetrahedron. And then with four copies of the larger tetrahedron, we make an even larger one. We stop there though, because 16 tetrahedra is enough to get the picture.
I'm also inspired by Tomoko Fuse's Unit Origami, where she shows ways to connect simple polyhedra together. But what larger whole can I construct with lots of little polyhedra? And so came the idea of the Sierpinski Tetrahedron. All that remained was to design a connector so that the tetrahedra would stick together. I'm not entirely satisfied with the connector design though, since it could stand to be more stable.
I tried searching for other connector solutions on the internet, and I found that most people just use tape. Except for this one, which claims to use paper links.
From Michal Kosmulski
But as I can plainly see, there are no connectors. I think the website is lying to me. You can't fool an origamist!











0 comments:
Post a Comment